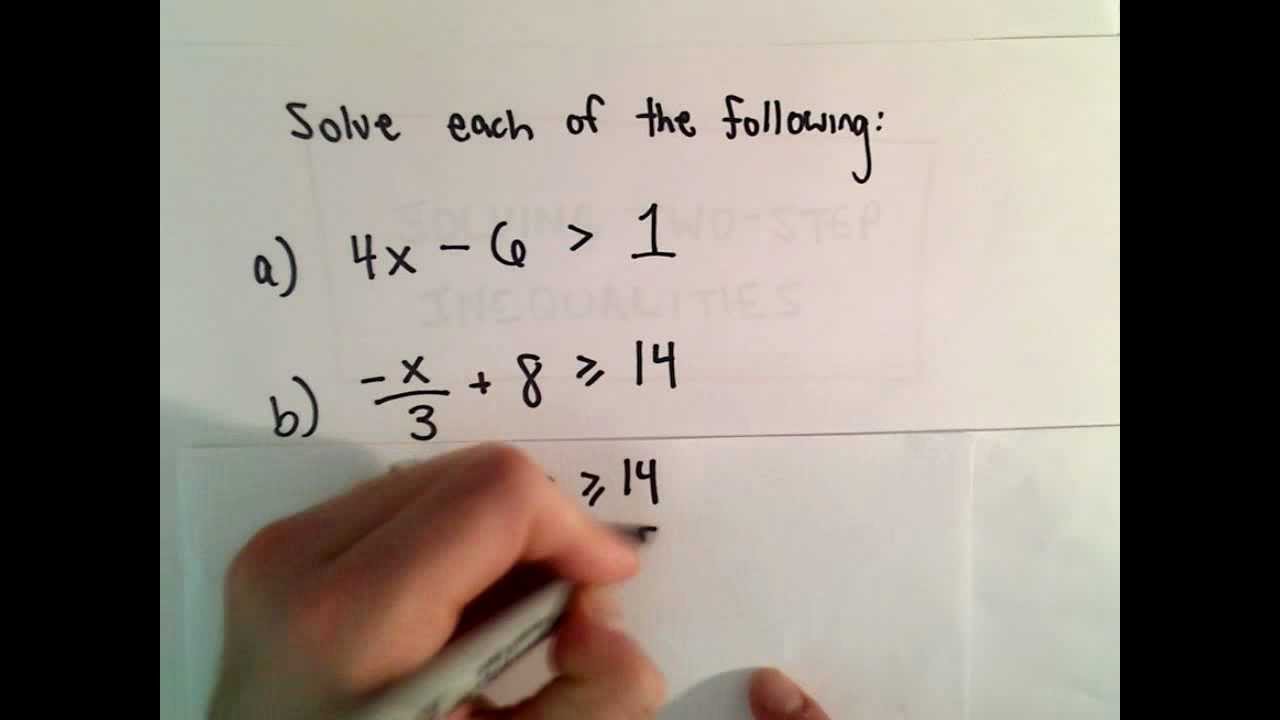Designing instructional experiences for students that are inclusive, engaging and effective take thoughtful planning, adjusting and reflecting on the part of the teacher. Over the course of your educational career, you will see different types of lesson design processes, templates and formats that have many types of criteria. Some of the criteria included on these templates might be: reading strategies, assessments, warm-ups, discussion prompts, differentiated writing tasks, language supports, real life connections, and the list goes on and on. What will remain true throughout, is the focus you will place on differentiating, empowering and setting each student up for content and literacy success in your classroom. Pedro Noguera states, “That’s at the core of equity: understanding who your kids are and how to meet their needs. You are still focused on outcomes, but the path to get there may not be the same for each one.” While designing learning experiences, think about how the students in your class can feel valued and celebrated, while learning both of the content and literacy goals you have set up.
This week, we will think about the students in our future classroom and how to design learning experiences that support each child where they are while providing them with the support needed to achieve content and literacy growth. In our last course, we learned about the different stages in a learning map, and now we will begin implementing that learning! Our learning map process starts now, and keep in mind that in Week 3 we will have an opportunity to implement our completed learning map with a group of students!
Instructions
Resources
Review the following resources before you begin your assignment, as they will inform your work.
Read
- Teaching Content-Area Literacy and Disciplinary Literacy
- How Important is Teaching Literacy in All Content Areas?
- Planning for the Inclusive Classroom: Meeting the Needs of Diverse Learners by Alison Gould and Sharon Vaughn.
- Literacy in the Inclusive Classroom
Watch
ITL 520 – Learning Map Stage One
CCSS.ELA-Literacy (be sure to not only cite the standard reference, but to articulate the exact wording from the standard
CCSS.MATH.CONTENT.HSA.REI.A.1: Explain each step in solving a simple equation as following from the equality of numbers asserted at the previous step, starting from the assumption that the original equation has a solution.
CCSS.MATH.CONTENT.HSA.REI.B.3: Solve linear equations and inequalities in one variable, including equations with coefficients represented by letters.
CCSS.MATH.CONTENT.HSA.REI.C.6: Solve systems of linear equations exactly and approximately (e.g., with graphs), focusing on pairs of linear equations in two variables.
CCSS.MATH.CONTENT.HSA.REI.D.12: Graph the solutions to a linear inequality in two variables as a half-plane (excluding the boundary in the case of a strict inequality), and graph the solution set to a system of linear inequalities in two variables as the intersection of the corresponding half-planes.
CCSS.ELA-LITERACY.RST.11-12.3: Follow precisely a complex multistep procedure when carrying out experiments, taking measurements, or performing technical tasks; analyze the specific results based on explanations in the text.
CCSS.ELA-LITERACY.RST.11-12.9: Synthesize information from a range of sources (e.g., texts, experiments, simulations) into a coherent understanding of a process, phenomenon, or concept, resolving conflicting information when possible.
CA Content Standard(s)
Alignment to CA Content Standards not required this time, though always recommended
ELD Standard
Alignment to ELD Standards not required this time, though always recommended
Prior Knowledge
What do students have to know coming into your lesson? Think in terms of instructional academic language and vocabulary.
Students must have a solid understanding of basic algebraic concepts to succeed in this lesson. An understanding of variables and constants, as well as the ability to evaluate expressions, is required. These fundamental algebraic principles provide a solid foundation for learning more advanced topics.
Knowing inequality symbols is a crucial component of preparedness. Students are expected to know symbols such as < (less than), > (greater than), ≤ (less than or equal to), and ≥ (greater than or equal to). For more complex problem-solving, this proficiency is essential for interpreting and manipulating inequalities.
Based on this, students should be able to solve simple equations, particularly linear equations. Inequalities involving multiple steps will require isolating variables and inverse operations, essential skills that will be applied in multi-step inequalities.
Students are also encouraged to bring their knowledge of number line representation to the lesson. Familiarity with illustrating inequalities on a number line enhances understanding of numerical values relationships.
A solid grasp of math operations, including addition, subtraction, multiplication, and division, is a prerequisite. Additionally, students are expected to apply these operations to solve equations and inequalities, showing their ability to solve problems mathematically.
A basic understanding of the coordinate plane is assumed for this lesson. Students need to be familiar with the Cartesian coordinate plane and understand how inequalities relate to regions on this plane. A spatial understanding is essential for presenting and interpreting solutions graphically.
Mathematical vocabulary plays a crucial role in preparedness. Key terms such as coefficient, constant, solution, expression, and equation are expected to be understood by students. It is also vital to know terms related to inequalities, such as inequality statements, solution sets, and interval notations. This is to communicate and solve inequality problems effectively.
Success in the lesson depends on critical thinking and problem-solving skills. Emphasizing the importance of checking solutions for validity, students are encouraged to apply these skills to real-world scenarios. It enhances their ability to deal with complex mathematical problems through a reflective, analytical approach.
The importance of effective language skills cannot be overstated. Students should be able to read and interpret mathematical statements and problems. In addition, students must communicate their mathematical reasoning both orally and in writing, fostering a comprehensive and articulate understanding of concepts.
The ability to reason logically is a cornerstone of mathematical proficiency. To solve mathematical problems, students must follow logical steps and articulate their reasoning. Through an understanding of the underlying logic behind mathematical operations and how they relate to inequalities, a deeper understanding of the subject matter will be gained.
Big Question(s)
Your Learning Target Question(s)
- Understanding Concepts:
- What are the main differences between equations and inequalities?
- How do you represent inequalities on a number line?
- Solving Techniques:
- What strategies can you employ to solve multi-step inequalities?
- How do you isolate the variable in a complex inequality?
- Graphical Interpretation:
- How is the solution to a multi-step inequality shown on a coordinate plane?
- Can you interpret the graphical representation of a solution set in real-world contexts?
- Reasoning and Justification:
- Why is it crucial to verify the solutions of a multi-step inequality?
- How do you justify each step in solving a multi-step inequality?
- Real-World Application:
- When might you use a multi-step inequality to model a real-world problem?
- How do you translate a real-world scenario into a multi-step inequality and solve it?
- Communication Skills:
- How would you explain your solution process to a peer struggling with multi-step inequalities?
- Can you clearly express your reasoning in both written and oral forms?
- Connections to Prior Knowledge:
- How does solving multi-step inequalities build upon your understanding of basic algebraic concepts?
- In what ways are the skills learned in this lesson useful in other areas of math or real-life situations?
- Reflection on Learning:
- What challenges did you face in solving multi-step inequalities, and how did you overcome them?
- How has your comprehension of inequalities evolved through this lesson?
Concepts
The content we want students to learn, evaluate, and apply.
In a lesson on multi-step inequalities, students explore several core concepts essential to their understanding, evaluation, and application. The first step is to understand multi-step inequalities. Students must understand that multiple operations are employed to isolate the variable. This understanding forms the foundation for applying the concept to various mathematical and real-world scenarios.
The topic emphasizes recognizing and interpreting inequality symbols such as <, >, ≤, and ≥. By identifying these symbols, students can express relationships between quantities, a fundamental skill in working with inequalities. As students move beyond symbols, they must develop effective strategies for solving multi-step inequalities. By mastering inverse operations and combining like terms, students can solve various problems confidently.
This lesson’s graphic representation emphasizes the connection between solutions and the coordinate plane. Students need to be able to interpret how the solutions to inequalities manifest graphically in real-world contexts. Students are also challenged to recognize situations where multi-step inequalities are effective models in real-world situations. The ability to translate real-world problems into mathematical expressions and then solve them demonstrates the practicality of mathematics.
Solving multi-step inequalities requires logical reasoning and justification. For each step in the solution process, students must follow logical steps and provide clear reasoning. Another critical concept is effective communication of solutions, requiring students to articulate their thoughts orally and in writing. The process of peer explanation fosters collaborative learning and reinforces understanding through shared discourse.
A critical habit emphasized in this lesson is checking solutions for validity. Students are taught to identify and correct errors in their solution processes as part of their understanding of the importance of verifying solutions. Throughout the lesson, students are encouraged to recognize how multi-step inequalities build upon foundational algebraic concepts, allowing them to connect with prior knowledge. Transferability of knowledge is an essential element of a holistic mathematical education.
The final purpose of the lesson is to promote metacognition by encouraging reflection on learning. As students reflect on their learning journeys, they are encouraged to identify challenges and areas for improvement. As a result of this reflective process, students can improve their understanding of inequalities and enhance their problem-solving ability.
Skills
What skills do you want students to master?
- Algebraic Manipulation:
- Objective: Master the manipulation of algebraic expressions and equations.
- Mastery: Apply algebraic techniques to isolate variables and simplify expressions within multi-step inequalities.
- Symbolic Representation:
- Objective: Develop the ability to interpret and manipulate inequality symbols.
- Mastery: Proficiently work with symbols such as <, >, ≤, and ≥ to represent relationships between quantities.
- Equation Solving Techniques:
- Objective: Proficiency in solving linear equations.
- Mastery: Apply inverse operations and isolate variables effectively when solving basic linear equations within multi-step inequality problems.
- Graphical Understanding:
- Objective: Understand the graphical representation of inequalities on a coordinate plane.
- Mastery: Graphically represent and interpret solutions, recognizing how graphical representations align with algebraic solutions.
- Arithmetic Operations:
- Objective: Demonstrate proficiency in basic arithmetic operations.
- Mastery: Apply addition, subtraction, multiplication, and division accurately, particularly when working through multi-step inequalities.
- Coordinate Plane Proficiency:
- Objective: Navigate and understand the Cartesian coordinate plane.
- Mastery: Apply knowledge of the coordinate plane to interpret solutions and understand the relationship between inequalities and regions on the plane.
- Vocabulary Mastery:
- Objective: Comprehend and use mathematical vocabulary related to inequalities.
- Mastery: Understand terms such as coefficient, constant, solution, expression, inequality statement, solution set, and interval notation within multi-step inequalities.
- Critical Thinking in Problem Solving:
- Objective: Develop critical thinking skills when approaching mathematical problems.
- Mastery: Apply logical reasoning and critical thinking to analyze, strategize, and solve complex multi-step inequalities.
- Logical Reasoning in Mathematical Operations:
- Objective: Understand and apply logical reasoning to mathematical operations.
- Mastery: Articulate the logical steps in solving mathematical problems, especially within multi-step inequalities.
- Verification and Error Analysis:
- Objective: Develop the habit of verifying solutions and identifying errors.
- Mastery: Systematically check the validity of solutions, recognize errors, and correct them as part of the problem-solving process.
Task
List both teacher actions (TA) and student actions (SA) for each skill
- Algebraic Manipulation:
- TA: Provide clear explanations and demonstrations of algebraic manipulation techniques.
- SA: Practice isolating variables and simplifying expressions through guided exercises and examples.
- Symbolic Representation:
- TA: Teach and reinforce the meaning and application of inequality symbols.
- SA: Practice interpreting and using inequality symbols in various mathematical contexts.
- Equation Solving Techniques:
- TA: Present strategies for solving linear equations, emphasizing inverse operations.
- SA: Apply inverse operations to solve basic linear equations, progressing to multi-step inequalities.
- Graphical Understanding:
- TA: Demonstrate how to represent inequalities on a coordinate plane graphically.
- SA: Graph solutions to inequalities, interpreting the graphical representation with algebraic solutions.
- Arithmetic Operations:
- TA: Reinforce the proper application of addition, subtraction, multiplication, and division.
- SA: Apply arithmetic operations accurately when solving multi-step inequalities.
- Coordinate Plane Proficiency:
- TA: Teach the basics of navigating and understanding the Cartesian coordinate plane.
- SA: Interpret solutions and identify regions on the coordinate plane related to inequalities.
- Vocabulary Mastery:
- TA: Define and review key mathematical terms related to inequalities.
- SA: Use and apply the defined vocabulary in explanations and problem-solving.
- Critical Thinking in Problem Solving:
- TA: Pose challenging problems that require critical thinking skills.
- SA: Analyze and strategize solutions for complex multi-step inequalities, engaging in discussions about problem-solving approaches.
- Logical Reasoning in Mathematical Operations:
- TA: Encourage students to articulate the logic behind their solution steps.
- SA: Clearly communicate the logical steps to solve mathematical problems, especially within multi-step inequalities.
- Verification and Error Analysis:
- TA: Emphasize the importance of verifying solutions and identifying errors.
- SA: Develop a habit of systematically checking the validity of solutions and recognizing and correcting errors in the solution process.
Strategy
Identify the instructional strategy:
Instructional Strategy: Guided Practice with Real-World Applications
By the end of the lesson, students will have acquired a solid understanding of multi-step inequalities and can apply their knowledge to real-world scenarios. The instructional strategy employs guided practice focusing on real-world applications to ensure students can transfer their learning to practical situations.
Performance (Verb)
List the verbs using Blooms or DOK:
- Blooms
- Remembering
- Understanding
- Applying
- Analyzing
- Creating
- DOK
- Understanding
- Applying
- Analyzing
- Creating
Condition
Describe the circumstances under which the performance takes place. Include: 1. Tools/Resources/Supports (what students will or will not use) 2. Environment (where the performance takes place).
Allowed Tools:
- Pencils, erasers, and graphing paper for solving mathematical problems.
- Calculators or other computational tools as deemed appropriate for the specific lesson.
- Reference materials, including a glossary of mathematical terms and a list of inequality symbols.
Environment:
- Classroom Setting:
- The performance is conducted within the familiar classroom environment.
- Students have individual desks or workspaces arranged to minimize distractions and facilitate concentration.
- Access to Technology:
- Availability of technology for interactive graphing activities, potentially including computers or tablets.
- An interactive whiteboard may be utilized for collaborative discussions and demonstrations.
- Teacher Guidance:
- The teacher circulates the classroom, providing support and clarification as needed.
- Peer collaboration is encouraged, with students able to engage in discussions and share solution strategies during designated collaboration periods.
Criterion: How will you measure student learning?
Describe what the criterion is:
- Accuracy of Solutions: The correctness of the solutions provided by the students for both algebraic and graphical representations of multi-step inequalities.
- Logical Reasoning: The ability of students to articulate the logical steps taken in solving mathematical problems, especially within the context of multi-step inequalities.
- Application to Real-World Scenarios: The proficiency with which students translate real-world scenarios into multi-step inequalities, solve them, and interpret the solutions in practical contexts.
- Graphical Representation: The accuracy of graphical representations on a coordinate plane, including the correct identification of solution regions and the alignment with algebraic solutions.
- Vocabulary and Symbol Usage: The appropriate use of mathematical vocabulary related to inequalities, such as coefficient, constant, solution, expression, and the accurate application of inequality symbols.
- Efficiency and Time Management: For timed assessments, the efficiency and time management skills demonstrated by students in solving multi-step inequalities within the allocated time frame.
- Problem-Solving Strategies: The effectiveness and variety of problem-solving strategies students apply when faced with complex multi-step inequality problems.
- Verification and Error Analysis: The ability to systematically check a solution’s validity and identify and correct errors in the solution process.
- Collaborative Engagement: The extent to which students actively engage in peer discussions, share solution strategies, and collaboratively work through selected problems.
- Overall Understanding: A holistic evaluation of students’ understanding of multi-step inequalities, including connecting different concepts and applying them across various scenarios.
Write a Learning Objective
Students will in (environment) be able to (verb) by using the strategy of _______________, with the support of (tools/resources) with (speed or accuracy) as measured by (how will you record the evidence of student learning).
Students will, in a collaborative classroom setting, be able to solve multi-step inequalities by applying algebraic manipulation techniques, graphically representing solutions on a coordinate plane, and interpreting the graphical representations, with the support of pencils, erasers, and graphing paper, and calculators with accuracy as measured by a combination of completed problem sets, graphical representations, and a brief written reflection on the problem-solving process.
Student Learning Target
I can statement:
I can solve multi-step inequalities, graph the solutions on a coordinate plane, and interpret the graphical representations with accuracy and clear articulation of logical solution steps.
Social and Emotional Learning Strategies
- Positive Behavior Reinforcement: Use positive reinforcement strategies to acknowledge and celebrate students’ social and emotional growth, fostering a positive classroom culture
- Mindfulness Practices: Integrate mindfulness exercises or short meditation sessions into the daily routine to help students develop self-awareness, focus, and stress management skills.
- Collaborative Learning: Incorporate collaborative learning activities to enhance interpersonal skills, teamwork, and relationship-building among students. This encourages communication and cooperation.
- Goal Setting: Engage students in setting both academic and personal goals. Goal-setting fosters a sense of purpose, motivation, and resilience, contributing to students’ overall well-being and success.
- Positive Behavior Reinforcement: Use positive reinforcement strategies to acknowledge and celebrate students’ social and emotional growth, fostering a positive classroom culture.
Student Misconceptions
- Inequality Direction Confusion: Misinterpreting the direction of the inequality symbol, such as reversing the meaning of “<” and “>.”
- Incorrect Application of Inverse Operations: Misapplying inverse operations when solving multi-step inequalities, leading to errors in isolating variables.
- Graphical Misinterpretation: Misinterpreting the graphical representation of inequalities on a coordinate plane, such as inaccurately identifying solution regions.
- Confusion with Compound Inequalities: Difficulty in understanding and solving compound inequalities involving multiple inequalities combined with “and” or “or” statements.
- Neglecting the Importance of Checking Solutions: Overlooking the necessity of checking solutions for validity after solving multi-step inequalities.
- Error in Translating Real-World Scenarios: Difficulty in translating real-world problems into accurate multi-step inequalities.
- Overreliance on Memorization: Relying on memorization of steps rather than understanding the underlying concepts of multi-step inequalities.
- Incomplete Understanding of Interval Notation: Incomplete or inaccurate understanding of interval notation and its application in representing solution sets.